Digikam/AspectRatios/it: Difference between revisions
Importing a new version from external source |
Importing a new version from external source |
||
| Line 8: | Line 8: | ||
Ecco come farli. | Ecco come farli. | ||
Scegli l'immagine e premi <keycap>F4</keycap> o fai clic destro sull'immagine e scegli <menuchoice>Modifica</menuchoice>. Nella finestra dell'editor vai sulla barra del menu e fai clic su <menuchoice>Trasforma -> Taglia alle proporzioni</menuchoice>, comparirà questa finestra. | |||
Revision as of 15:39, 22 February 2011
Effettuare tagli secondo proporzioni in digiKam
Trascritto dal blog di Mohamed Malik, 21 febbraio 2011
Ogni singolo schermo ha una proporzione, cioè un rapporto tra la larghezza e l'altezza. DigiKam può effettuare tagli conformi a queste molto facilmente. È molto utile se stai creando sfondi ed immagini che debbano adattarsi ad una determinata proporzione. Personalmente utilizzo questa funzionalità quando carico i miei sfondi su kde-look.
Ecco come farli.
Scegli l'immagine e premi F4 o fai clic destro sull'immagine e scegli . Nella finestra dell'editor vai sulla barra del menu e fai clic su , comparirà questa finestra.
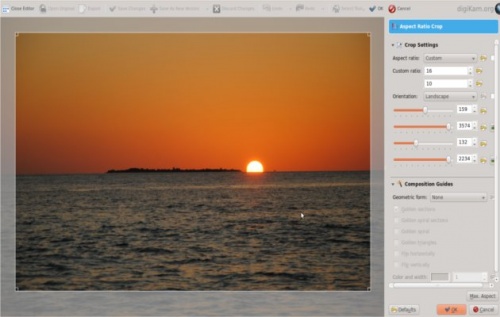
The tool has the following predefined ratios:
- 1:1
- 3:2
- 4:3
- 5:4
- 7:5
- 10:7 & 5:8
It also has an option named golden aspect ratio… I still havent figured out what it does or what it means! [1]
It also gives the option of custom aspect ratio. Therefore you can choose any ratio that you may prefer.
After choosing an aspect ratio hold the tiny square that you see on the edges of the highlighted area and move to the area of the image that you want to crop any area you select will have the aspect that you specified.
References
- ↑ An explanation of the Golden Ratio can be found at the Maths is Fun site
