KmPlot/Using Sliders/de: Difference between revisions
< KmPlot
Pipesmoker (talk | contribs) (Created page with "KmPlot/Einsatz von Schiebereglern") |
Pipesmoker (talk | contribs) (Created page with "Ein besonderes Merkmal von '''KmPlot''' ist die Veranschaulichung der Wirkung eines Parameters auf den Kurvernverlauf einer Funktion.") |
||
| Line 1: | Line 1: | ||
<languages /> | <languages /> | ||
Ein besonderes Merkmal von '''KmPlot''' ist die Veranschaulichung der Wirkung eines Parameters auf den Kurvernverlauf einer Funktion. | |||
==Moving a Sinus Curve== | ==Moving a Sinus Curve== | ||
Revision as of 17:52, 11 October 2010
Ein besonderes Merkmal von KmPlot ist die Veranschaulichung der Wirkung eines Parameters auf den Kurvernverlauf einer Funktion.
Moving a Sinus Curve
Let's see, how to move a sinus curve left and right:
- Create a new cartesian plot.
- Enter the equation
f(x,a) = sin(x-a)
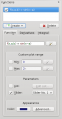
- Check the option and choose from the drop down list.
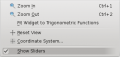
- To make the available sliders visible, check
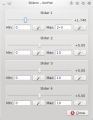
Now you can move the slider and see how the parameter value modifies the position of the curve.
- Screenshots
-
Input
-
Show sliders option
-
Slider window
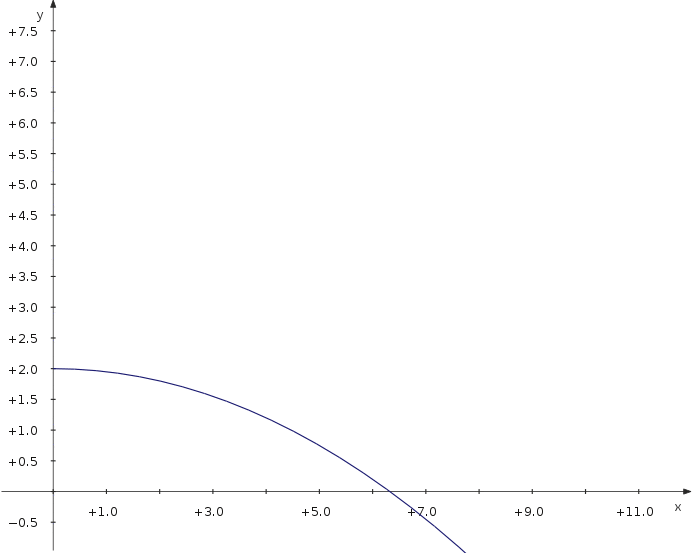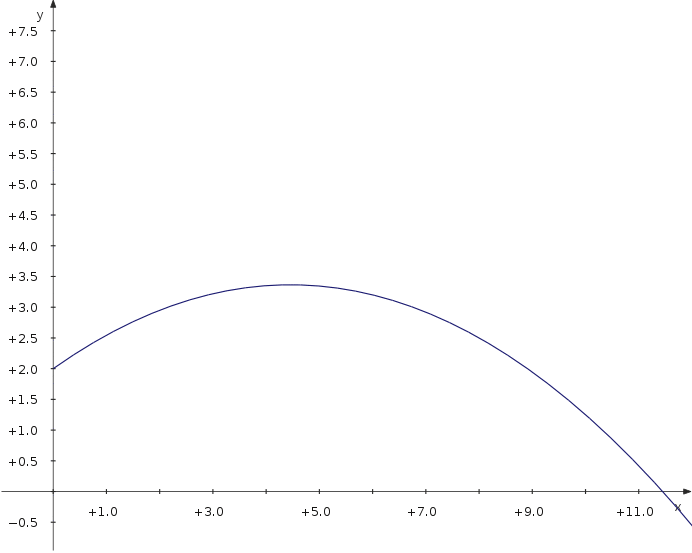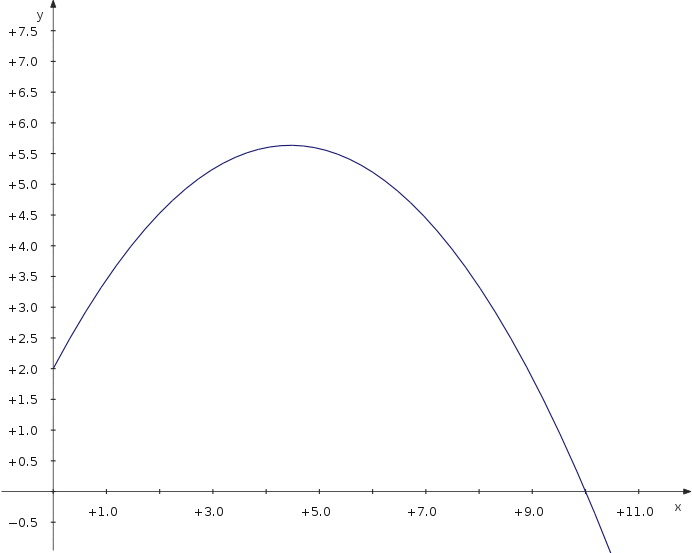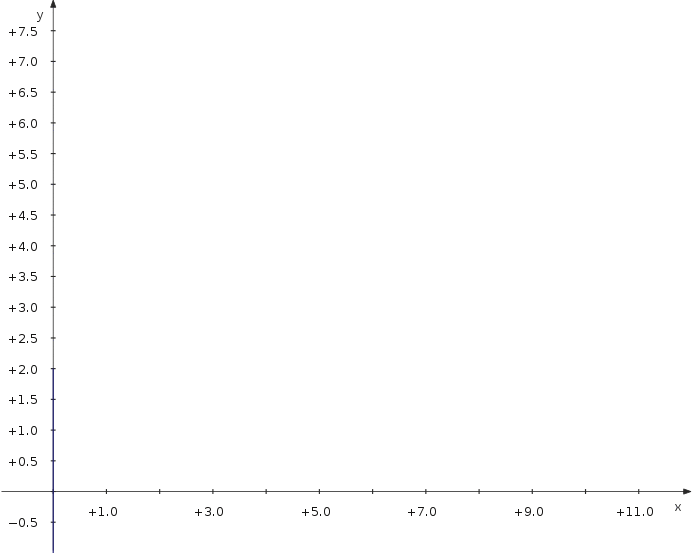
Trajectory of a Projectile
Now let's have a look at the maximum distance of a projectile thrown with different angles. We use a parametric plot depending on an additional parameter which is the angle.
- Define a contant v_0 for the starting velocity.
- Create a new parametric plot
- Enter the equations
f_x(t,α) = v_0∙cos(α)∙t f_y(t,α) = 2+v_0∙sin(α)∙t−5∙t^2
- Check the option and choose from the drop down list.
- To make the available sliders visible, check
Now you can move the slider and see how the distance depends on the parameter value.